Main research interests & current projects
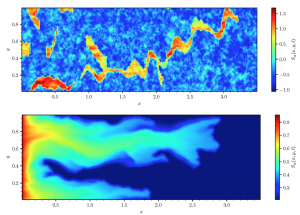
EOR using foam (SAG)
Mathematical and computational modeling of foam injection as an enhanced oil recovery technique applied to Brazil pre-salt reservoirs.
It is an international collaboration between UFJF (Federal University of Juiz de Fora, Brazil), TU Delft (Delft University of Technology, Holland) and PUC-Rio (Pontifical Catholic University of Rio de Janeiro, Brazil) supported by Shell Brazil
The main objectives of this 4-year project are:
- Improve the understanding of physics and mathematics describing the foam displacement in porous media as an enhanced oil recovery (EOR) technique applied to Brazil pre-salt reservoirs.
- Develop analytic and numerical methods that can be used to test and validate commercial simulators.
The project has a strongly multidisciplinary appeal bringing together the mathematical analysis and numerical simulations performed in UFJF, experimental investigation realized in PUC-Rio and physical modeling assisted by renowned experts in foam EOR from TU Delft.
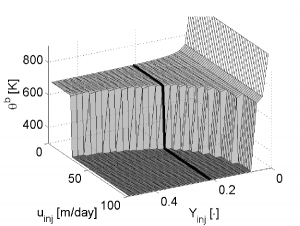
Thermal EOR
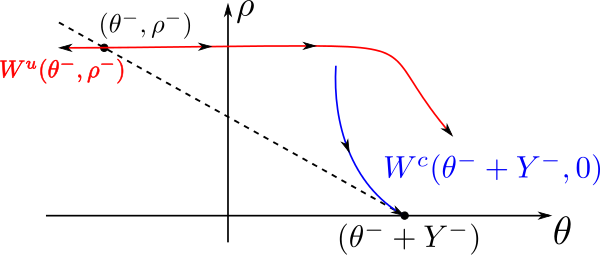
Combustion traveling waves
Filtration combustion is a thermal technique with great potential for use in the exploration of offshore oil, as in the case of the pre-salt reservoirs. The modeling of combustion in porous media involves Fluid Dynamics and Chemical Kinetics. The models describing in-situ combustion are composed of reaction-convection-diffusion equations, present different scales (stiff problems), and are difficult to solve both mathematically and computationally.
This project is focused on Applied Mathematics and proposes to develop research in the intersection between areas of Partial Differential Equations and Dynamical Systems aiming at applications in Petroleum Engineering.
Thermal flows in Porous Media
The aim of this project consists in modeling complex physical phenomena in a simple way in order to make analytical techniques possible to be applied. Two interesting problems that are studied following this idea are: (1) Recovery of shale gas by using combustion and (2) Microwave stimulated water flooding.
Population dynamics
The global incidences of Dengue and, more recently, Zica virus have increased the interest in studying and understanding the mosquito population dynamics. This project is based on the study of nonlinear mathematical models dealing with the life cycle of the dengue mosquito using partial differential equations.
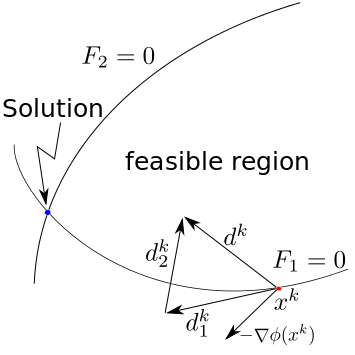
Inverse problems, Regularization, and Optimization
Parabolic-type problems, involving a variational complementarity formulation, arise in mathematical models of several applications in Engineering, Economy, Biology, and different branches of Physics. These kinds of problems present several analytical and numerical difficulties related, for example, to time evolution and a moving boundary. We develop numerical methods that employ a global convergent nonlinear complementarity (or mixed complementarity) algorithms for solving a discretized problem at each time step. Space discretization is implemented using the finite difference implicit scheme and the finite element method.
Some interesting topics involve Regularization techniques and Inverse Problems.
Others
Some collaboration works.
Related papers